「ミラー対称性 (弦理論)」の版間の差分
Enyokoyama (会話 | 投稿記録) →ミラー対称性の発見: 研究者の名前を記載した、Philip Candelas, Monika Lynker, and Rolf Schimmrigk |
Enyokoyama (会話 | 投稿記録) →参考文献: 文献修正、英語版に以前あり削除されたものも残しました.(複素幾何学、シンプレクティック幾何学の記載が英語版では削除) |
||
| 215行目: | 215行目: | ||
==参考文献== |
==参考文献== |
||
==References== |
|||
{{refbegin|2}} |
{{refbegin|2}} |
||
* {{cite book |editor1-first=Paul |editor1-last=Aspinwall |editor2-first=Tom |editor2-last=Bridgeland |editor3-first=Alastair |editor3-last=Craw |editor4-first=Michael |editor4-last=Douglas |editor5-first=Mark |editor5-last=Gross |editor6-first=Anton |editor6-last=Kapustin |editor7-first=Gregory |editor7-last=Moore |editor8-first=Graeme |editor8-last=Segal |editor9-first=Balázs |editor9-last=Szendröi |editor10-first=P.M.H. |editor10-last=Wilson |title=Dirichlet Branes and Mirror Symmetry |year=2009 |publisher=American Mathematical Society | isbn=978-0-8218-3848-8}} |
* {{cite book |editor1-first=Paul |editor1-last=Aspinwall |editor2-first=Tom |editor2-last=Bridgeland |editor3-first=Alastair |editor3-last=Craw |editor4-first=Michael |editor4-last=Douglas |editor5-first=Mark |editor5-last=Gross |editor6-first=Anton |editor6-last=Kapustin |editor7-first=Gregory |editor7-last=Moore |editor8-first=Graeme |editor8-last=Segal |editor9-first=Balázs |editor9-last=Szendröi |editor10-first=P.M.H. |editor10-last=Wilson |title=Dirichlet Branes and Mirror Symmetry |year=2009 |publisher=American Mathematical Society | isbn=978-0-8218-3848-8}} |
||
* {{cite journal |last=Candelas |first=Philip |last2=de la Ossa |first2=Xenia |last3=Green |first3=Paul |last4=Parks |first4=Linda |year=1991 |title=A pair of Calabi–Yau manifolds as an exactly soluble superconformal field theory |journal=Nuclear Physics B |volume=342 |issue=1 |pages=21–74 |doi=10.1007/s00220-007-0354-8|bibcode=2007CMaPh.276..671W }}{{failed verification|date=May 2014}} |
|||
* {{cite journal | |
* {{cite journal |last1=Candelas |first1=Philip |last2=Horowitz |first2=Gary |last3=Strominger |first3= Andrew |last4=Witten |first4=Edward |year=1985 |title=Vacuum configurations for superstrings |journal=Nuclear Physics B |volume=258 |issue= |pages=46–74|bibcode = 1985NuPhB.258...46C |doi=10.1016/0550-3213(85)90602-9 }} |
||
| ⚫ | * {{cite journal |last1=Candelas |first1=Philip |last2=Lynker |first2=Monika |last3=Schimmrigk |first3=Rolf |year=1990 |title=Calabi–Yau manifolds in weighted <math>\mathbb{P}_4</math> |journal=Nuclear Physics B |volume=341 |issue=1 |pages=383–402 |doi=10.1016/0550-3213(90)90185-G|bibcode=1990NuPhB.341..383C }} |
||
* {{cite journal |last1= |
* {{cite journal |last1=Dixon |first1=Lance |year=1988 |title=Some world-sheet properties of superstring compactifications, on orbifolds and otherwise |journal=ICTP Ser. Theoret. Phys. |volume=4 |pages=67–126 |isbn=978-9971-5-0452-6}} |
||
* {{cite journal |last1=Givental |first1=Alexander |year=1996 |title=Equivariant Gromov-Witten invariants |journal=International Mathematics Research Notices |volume=1996 |issue=13 |pages=613–663 |doi=10.1155/S1073792896000414}} |
|||
* {{cite journal |last1= |
* {{cite journal |last1=Givental |first1=Alexander |year=1998 |title=A mirror theorem for toric complete intersections |journal=Topological field theory, primitive forms and related topics |pages=141–175 |doi=10.1007/978-1-4612-0705-4_5|isbn=978-1-4612-6874-1 }} |
||
| ⚫ | |||
| ⚫ | |||
| ⚫ | * {{cite book |editor1-first=Kentaro |editor1-last=Hori |editor2-first=Sheldon |editor2-last=Katz |editor3-first=Albrecht |editor3-last=Klemm |editor4-first=Rahul |editor4-last=Pandharipande |editor5-first=Richard |editor5-last=Thomas |editor6-first=Cumrun |editor6-last=Vafa |editor7-first=Ravi |editor7-last=Vakil |editor8-first=Eric |editor8-last= Zaslow|title=Mirror Symmetry |year=2003 |publisher=American Mathematical Society|url=http://math.stanford.edu/~vakil/files/mirrorfinal.pdf|isbn=0-8218-2955-6}} |
||
* {{cite arXiv |last1=Hori |first1=Kentaro |last2=Vafa |first2=Cumrun |eprint=hep-th/0002222 |title=Mirror Symmetry |year=2000}} |
|||
| ⚫ | |||
| ⚫ | |||
* {{cite journal |last=Kontsevich |first=Maxim |date=1995a |publisher=Birkhäuser |doi=10.1007/978-1-4612-4264-2_12|chapter=Enumeration of Rational Curves Via Torus Actions |title=The Moduli Space of Curves |isbn=978-1-4612-8714-8 |pages=335 }} |
|||
* {{cite journal |last1=Kontsevich |first1=Maxim |year=1995b |title=Homological algebra of mirror symmetry |journal=Proceedings of the International Congress of Mathematicians |pages=120–139|bibcode=1994alg.geom.11018K |arxiv=alg-geom/9411018 }} |
|||
| ⚫ | * {{cite journal |last1=Lerche |first1=Wolfgang |last2=Vafa |first2=Cumrun |last3=Warner |first3=Nicholas |year=1989 |title=Chiral rings in <math>N = 2</math> superconformal theories |journal=Nuclear Physics B |volume=324 |issue=2 |pages=427–474|bibcode = 1989NuPhB.324..427L |doi = 10.1016/0550-3213(89)90474-4 }} |
||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
* {{cite journal |last1=Lian |first1=Bong |last2=Liu |first2=Kefeng |last3=Yau |first3=Shing-Tung |year=2000 |title=Mirror principle, IV |journal=Surveys in Differential Geometry |pages=475–496|bibcode=2000math......7104L |arxiv=math/0007104 }} |
|||
* {{cite book |last1=Mac Lane |first1=Saunders |title=Categories for the Working Mathematician |year=1998 |isbn=978-0-387-98403-2}} |
|||
| ⚫ | |||
* {{cite journal |last1=Sakai |first1=Norisuke |last2=Senda |first2=Ikuo |year=1986 |title=Vacuum energies of string compactified on torus |journal=Progress of Theoretical Physics |volume=75 |issue=3 |pages=692–705|bibcode = 1986PThPh..75..692S |doi = 10.1143/PTP.75.692 }} |
|||
| ⚫ | * {{cite journal |last1=Strominger |first1=Andrew |last2=Yau |first2=Shing-Tung |last3=Zaslow |first3=Eric |year=1996 |title=Mirror symmetry is T-duality |journal=Nuclear Physics B |volume=479 |issue=1 |pages=243–259|arxiv = hep-th/9606040 |bibcode = 1996NuPhB.479..243S |doi = 10.1016/0550-3213(96)00434-8 }} |
||
| ⚫ | |||
| ⚫ | |||
* {{cite journal |last1=Witten |first1=Edward |year=1990 |title=On the structure of the topological phase of two-dimensional gravity |journal=Nuclear Physics B |volume=340 |issue=2–3 |pages=281–332|bibcode = 1990NuPhB.340..281W |doi = 10.1016/0550-3213(90)90449-N }} |
|||
* {{cite journal |last1=Witten |first1=Edward |year=1992 |title=Mirror manifolds and topological field theory |journal=Essays on mirror manifolds |pages=121–160 |isbn=978-962-7670-01-8}} |
|||
| ⚫ | |||
* {{Cite book| last1=Zaslow | first1=Eric | contribution=Mirror Symmetry | year=2008 | title=The Princeton Companion to Mathematics | editor-last=Gowers | editor-first=Timothy | isbn=978-0-691-11880-2 }} |
|||
* {{cite book |last1=Zwiebach |first1=Barton |title=A First Course in String Theory |year=2009 |publisher=Cambridge University Press |isbn=978-0-521-88032-9}} |
|||
* {{cite journal |last1=Givental |first1=Alexander |year=1996 |title=Equivariant Gromov-Witten invariants |journal=International Mathematics Research Notices |volume=1996 |issue=13 |pages=613–663}} |
* {{cite journal |last1=Givental |first1=Alexander |year=1996 |title=Equivariant Gromov-Witten invariants |journal=International Mathematics Research Notices |volume=1996 |issue=13 |pages=613–663}} |
||
* {{cite journal |last1=Givental |first1=Alexander |year=1998 |title=A mirror theorem for toric complete intersections |journal=Topological field theory, primitive forms and related topics |pages=141–175}} |
* {{cite journal |last1=Givental |first1=Alexander |year=1998 |title=A mirror theorem for toric complete intersections |journal=Topological field theory, primitive forms and related topics |pages=141–175}} |
||
| ⚫ | |||
| ⚫ | * {{cite book |editor1-first=Kentaro |editor1-last=Hori |editor2-first=Sheldon |editor2-last=Katz |editor3-first=Albrecht |editor3-last=Klemm |editor4-first=Rahul |editor4-last=Pandharipande |editor5-first=Richard |editor5-last=Thomas |editor6-first=Cumrun |editor6-last=Vafa |editor7-first=Ravi |editor7-last=Vakil |editor8-first=Eric |editor8-last= Zaslow|title=Mirror Symmetry |year=2003 |publisher=American Mathematical Society|url=http://math.stanford.edu/~vakil/files/mirrorfinal.pdf|isbn= |
||
| ⚫ | * {{cite journal |last1=Intriligator |first1=Kenneth |last2=Seiberg | |
||
| ⚫ | |||
* {{cite journal |last=Kontsevich |first=Maxim |date=1995 |title=Enumeration of rational curves via torus actions |publisher=Birkhäuser}} |
|||
* {{cite journal |last1=Kontsevich |first1=Maxim |year=1995 |title=Homological algebra of mirror symmetry |journal=Proceedings of the International Congress of Mathematicians |pages=120–139}} |
* {{cite journal |last1=Kontsevich |first1=Maxim |year=1995 |title=Homological algebra of mirror symmetry |journal=Proceedings of the International Congress of Mathematicians |pages=120–139}} |
||
| ⚫ | * {{cite journal |last1= |
||
| ⚫ | |||
| ⚫ | |||
| ⚫ | |||
* {{cite journal |last1=Lian |first1=Bong |last2=Liu |first2=Kefeng |last3=Yau |first3=Shing-Tung |year=2000 |title=Mirror principle, IV |journal=Surveys in Differential Geometry |pages=475–496}} |
* {{cite journal |last1=Lian |first1=Bong |last2=Liu |first2=Kefeng |last3=Yau |first3=Shing-Tung |year=2000 |title=Mirror principle, IV |journal=Surveys in Differential Geometry |pages=475–496}} |
||
* {{cite book |last1=Mac Lane |first1=Saunders |title=Categories for the Working Mathematician |year=1998 |isbn=978-0387984032}} |
* {{cite book |last1=Mac Lane |first1=Saunders |title=Categories for the Working Mathematician |year=1998 |isbn=978-0387984032}} |
||
* {{cite journal| author=Moore, Gregory | title=What is... a Brane?| journal=Notices of the AMS| year=2005 | url=http://www.ams.org/notices/200502/what-is.pdf |format=PDF| accessdate=June 2013 |page=214| volume=52}} |
|||
| ⚫ | |||
| ⚫ | |||
| ⚫ | * {{cite journal |last1= |
||
| ⚫ | |||
| ⚫ | |||
* {{cite journal |last1=Witten |first1=Edward |year=1992 |title=Mirror manifolds and topological field theory |journal=Essays on mirror manifolds |pages=121–160}} |
* {{cite journal |last1=Witten |first1=Edward |year=1992 |title=Mirror manifolds and topological field theory |journal=Essays on mirror manifolds |pages=121–160}} |
||
| ⚫ | |||
* {{Citation | last1=Zaslow | first1=Eric | contribution=Mirror Symmetry | year=2008 | title=The Princeton Companion to Mathematics | editor-last=Gowers | editor-first=Timothy | isbn=978-0691118802}} |
* {{Citation | last1=Zaslow | first1=Eric | contribution=Mirror Symmetry | year=2008 | title=The Princeton Companion to Mathematics | editor-last=Gowers | editor-first=Timothy | isbn=978-0691118802}} |
||
| ⚫ | |||
{{refend}} |
{{refend}} |
||
2014年5月9日 (金) 11:29時点における版
数学や理論物理学では、ミラー対称性(mirror symmetry)はカラビ・ヤウ多様体(Calabi-Yau manifold)と呼ばれる幾何学的な対象の間の関係である。2つの カラビ・ヤウ多様体が、幾何学的には全く異なっているが、にもかかわらず、弦理論の隠れた次元としてそれらを扱うと、等価であることが起きうる対称性のことを言う。この場合、多様体は、互いに「ミラー多様体」であると呼ばれる。
ミラー対称性はもともとは、物理学者によって発見された。数学者はミラー対称性に興味を持ち始めたのは1990年ころである。1990年頃には、フィリップ・キャンデラス(Philip Candelas)、ゼニア・デ・ラ・オッサ(Xenia de la Ossa)、パウル・グリーン(Paul Green)、リンダ・パークス(Linda Parks)は、ミラー対称性を数々の方程式の解の数を数える数学の分野である数え上げ幾何学で使うことができることを示した。実際、キャンデラスたちは、ミラー対称性を使いカラビ・ヤウ多様体の上の有理曲線を数えることができ、長くにわたり未解決であった問題を解明できることを示した[1]。元来のミラー対称性へのアプローチは、理論物理学者からの数学的には厳密(mathematical rigor)ではないアイデアに基づいているにもかかわらず、数学者はミラー対称性予想のいくつかを数学的に厳密な証明(mathematical proof)に成功しつつある[2]。
今日では、ミラー対称性は純粋数学の主要な研究テーマであり、数学者は物理学者の直感に基づくミラー対称性を数学的に深く理解しつつある[3]。ミラー対称性は弦理論の計算を実行する際の基本的なツールでもある[4]。ミラー対称性への主要なアプローチは、マキシム・コンツェビッチ(Maxim Kontsevich)のホモロジカルミラー対称性予想のプログラムやアンドリュー・ストロミンジャー(Andrew Strominger)、シン=トゥン・ヤウ(Shing-Tung Yau)、エリック・ザスロフ(Eric Zaslow)のSYZ予想[5]を含んでいる。
オーバービュー
ミラー対称性のアイデア

物理学では、弦理論は、その中では素粒子を点状の粒子とは考えずに、弦と呼ばれる 1次元の対象で置き換えた理論的フレームワーク(theoretical framework)である。これらの弦は通常の弦のループや小さな区分のように見える。弦理論は、どのように弦が空間の中を伝搬するか互いに相互作用するかを記述する。弦のスケールよりも大きな距離スケールでは、弦は通常の粒子のように見え、質量や電荷を持ち、弦の振動状態によりきめられる他の性質を持っている。弦が分裂したり結合したりすることには、粒子の輻射や吸収が対応し、粒子の間の相互作用を惹き起す。[6]
弦理論の記述する世界と日常の世界の間には、確かに差異がある。日常生活では、3つ空間次元(上下、左右、前後)が存在し、1つの時間次元(以後以前)が存在する。このように、現代物理の言葉では、時空は 4次元である。[7] 弦理論の特別な有様の一つに、数学的な整合性のために時空の余剰次元(extra dimensions)を要求される。超弦理論である超対称性と呼ばれる理論上な考え方と協調する理論のバージョンでは、毎日の体験の中で慣れ親しんでいる 4次元に加えて、6次元の時空の余剰次元がある。[8]
弦理論の現在の研究の目標のひとつは、高エネルギー物理実験で観察される粒子を弦が再現するモデルを開発することである。観察と整合性を持たせるためには、そのような時空の次元は 4 である必要があるので、通常の距離スケールでは弦理論の余剰次元を消し去る方法を見つけなくてはならない。弦理論を基礎とする最も現実的なモデルでは、コンパクト化(compactification)と呼ばれる過程を通して行われる。[9]コンパクト化の考え方は、弦理論のある次元が円をなして自分で「閉じている」ようなものかもしれない。次元が巻きあがっている極限では、非常に小さくなり、有効理論ではより低い次元となっている理論を得る。このことの標準的な類似物は、庭のホースのような多次元の対象を考えることで、ホースを充分に遠い距離で見ると 1次元となり長さしか持っていないように見える。しかし、ホースに近づくと、第二の円周という次元を持っていることが分かる。このようにして、ホースの中の蟻は 2次元的に動くことができる。[10]

コンパクト化は、時空の有効次元が 4次元となるようなモデルを構成することに使うことができる。しかし、余剰次元をコンパクト化する全ての方法が、自然を記述する良い性質を持つモデルを作り出すわけではない。素粒子物理学で確認できるようなモデルを構成するためには、コンパクトな余剰次元はカラビ・ヤウ多様体の形をしている必要がある。[9]カラビ・ヤウ多様体は複雑な(典型では)6次元の形をしていて、あるテクニカルな条件を満たす。それらは、数学者のエウジェニオ・カラビ(Eugenio Calabi)とシン=トゥン・ヤウ(Shing-Tung Yau)の名前から命名された。[11]
1980年代後半、弦理論のそのようなコンパクト化をすると、対応するカラビ・ヤウ多様体が一意に再構成されることが可能ではないことが分かった。代わりに、2つのカラビ・ヤウ多様体が同じ物理を持つことが発見された。[12] これらの多様体はたがいに「ミラー」といわれる。全部の双対性はいまだ予想でしかないが、位相的弦理論の脈絡でのミラー対称性のバージョンがある。位相的弦理論はエドワード・ウィッテン[13] により導入された簡素化された弦理論のバージョンであり、このバージョンは数学者により厳密性(en:mathematical rigor)を持った。[14] 位相的弦理論の脈絡では、ミラー対称性は、2つの理論、A-モデルとB-モデルがある正確な意味で等価であることを言っている。[15]
弦理論のこれらのカラビ・ヤウコンパクト化が自然の正しい記述をもたらすかどうかは別として、異なるカラビ・ヤウ多様体の間のミラー対称性関係の存在は、重要な数学的結果である。[16] 弦理論に使われるカラビ・ヤウ多様体は、純粋数学的には興味深く、ミラー対称性はミラーカラビ・ヤウと同等な問題を解くことで数え上げ代数幾何学の多くの問題を数学者が解決できるようにした。[17] 今日、ミラー対称性は数学の研究の活発な領域であり、数学者たちは今も物理学者の直感に基づくミラー対称性の数学的理解を深めようと努力している。[18]
複素幾何学

ミラー双対の片側に現れる幾何学の一種を理解するためには、ここで複素平面の点を同一視することで、トーラス、ドーナツのようにひとつの穴のあいた閉曲面の構成を考える。このトーラスを構成するためには、最初に商 が実数ではない複素数のペア と を選ばねばならない. この最後の条件は、これらの点が一直線上にない(en:collinear)ことを確かなものとしている.従って、選択された点は、もう他の頂点が 0 と である平行四辺形を決定する。この平行四辺形の反対側の辺を同一視することで、求めるトーラスが得られる。
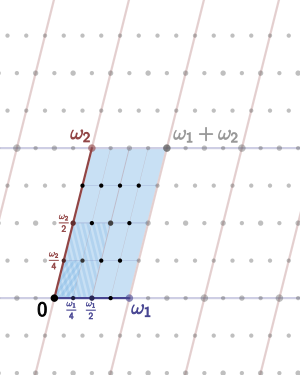
このようにして得られるトーラスは、ひとつのトーラスが他のトーラスと連続変形可能であるという意味ですべて同値である。[19] 他方、トーラスは加法構造を持っているので、区別することが可能となる。[20] すなわち、この方法で構成されたトーラスは、複素構造を持っていて、そのようなトーラス上の任意の点の近傍は複素平面の中にある領域のように見えることを意味する。
このトーラスの構成の中で、替わりに元のペアと共通因子(つまり、ある複素数 により と である)としてリスケールによって複素数のペア と が関連づけられるとすると、同値なトーラスを得る。従って、「比率」 でトーラス全体の集まりをパラメトライズすることはさらに便利である。この比率はリスケール によっては変わらない。一般性を失うことなしに、このパラメータ は正の虚部を持つので、 は上半平面に値を持つ。また、パラメータ , , と が同じトーラスに対応している。
もし 2つのトーラスがもともと異なる 値に対応しているとすると、それらは等価ではない複素構造を持つ。[21] パラメータ は、平行四辺形の対辺を同一視して構成されるトーラスの「形」として記述することができる。上で説明したように、ミラー対称性は 2つの物理学的な理論、位相的弦理論のA-モデルとB-モデルとを関連付ける。この双対性では、位相的B-モデルが時空の複素構造にのみ依存している。このようにして、もし「時空」がトーラスであるような理論を考えると、理論はパラメータ にのみ依存することになる。[22]
シンプレクティック幾何学
トーラスの幾何学のもう一つの側面は、トーラスのサイズである。さらに詳しくは、トーラスを単位四方形の対辺を同一視することにより得られる曲面としてみることができ、トーラスの面積はこの四辺形上の面積要素 で特定できる。単位四方形上の面積要素を積分することにより、対応するトーラスの面積 を得る。これらの概念を高次元にも一般化することができ、面積要素はシンプレクティック形式の考え方により一般化される。シンプレクティック形式を持つ空間の研究は、シンプレクティック幾何学と呼ばれる。[23]
ミラー対称性では、位相的弦理論のA-モデルが、時空のシンプレクティック幾何学に依存した理論である。その中では「時空」がトーラスである理論を考えると、A-モデルは連続的にパラメータ に依存する。[24]
T-双対
どのようにしてトーラスが複素平面の中の平行四辺形の対辺を同一視すると得ることができるかを見てみる。特別に単純例は、複素数 と それぞれが実軸と虚軸にある場合である。この場合には、 および と書くことができる。ここに と は実数である。上記の議論のようにして得られたトーラス上の複素構造は、数値 により特徴づけられる。
どのようにトーラスのシンプレクティック構造が面積要素により決定されるかを、すでに説明した。平行四辺形上の座標 と を、複素数により張られる平行四辺形の各々の辺が長さ 1 を持つように選ぶことができる。すると、このトーラスの面積要素は であり、単位正方形上で積分し となる。シンプレクティックパラメータ を積 と定義する。
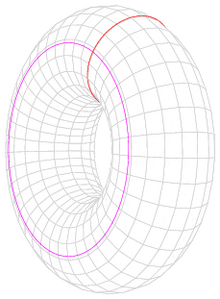
2つの円のカルテシアン積としてトーラスを考えることができることに注意する。このことは、トーラス(ピンクで表示されている)の赤道の各々の点に、経線の円(赤で表示されている)がある。
さて、トーラスは物理的理論での「時空」を表現することを想像すると、この理論の基本的な対象は、量子力学の規則に従い時空の中を伝搬する弦 (物理学)である。弦理論の双対性 (物理学)の一つにT-双対性がある。このことは、すべての一方での観測可能量は双対な記述での量と同一視されるという意味で、半径 の円の周りを伝搬する弦は、半径 の円の周りを伝搬する弦に等価となる。[25] 例えば、弦のある方向へでの運動量は、離散的な値をとり、弦が双対方向の円の周りへ何周り巻き付いているかを表す。[26] T-双対をトーラスの経線方向の円へ適用すると、別のトーラスにより表現される時空の中にある等価な記述が存在することがわかる。T-双対性は を へと変換し、この変換は と複素パラメータとシンプレクティックパラメータとを入れ替える。
一般の場合
一般にミラー対称性は、2つの物理理論の同値性であり、複素幾何学の問題をシンプレクティック幾何学の問題へ翻訳することでもある。ここで考えるトーラスは、単にコンパクトな(実)次元が 2 であるカラビ・ヤウ多様体であり、従って、ミラー対称性のもっとも単純な例である。[27] 弦理論への応用では、普通、6次元のカラビ・ヤウ多様体を考える。この 6次元は、時空の観測されえない次元に対応する。
上記の例の中のように、カラビ・ヤウ多様体は非常に変わった形をしているかもしれない。6-次元のカラビ・ヤウ多様体の形は、数学的にはある不変量(invariant)を使い記述される(不変量とは多様体に付随する数値であり、幾何学的に同じ多様体は同じ付随する数値を持つ)。例えば、カラビ・ヤウ多様体の形は大雑把にはオイラー標数と呼ばれる数値により記述され、ミラー双対のカラビ・ヤウ多様体は、(ペアの相手のカラビ・ヤウ多様体の)反対の符号をもつオイラー数となることができる。[28] 多くの異なって見える形が同じオイラー標数を持っていて、この不変量はカラビ・ヤウ多様体の形の単に粗い記述をするものでしかない。しかしながら、この粗さはベッチ数と呼ばれる数の和へとオイラー標数を分解することにより、詳しくすることができる[29]。さらに詳しくするためには、ホッジ数と呼ばれるミラーカラビ・ヤウ多様体の興味深い対称性を持っている不変量を使う[30]。
一般にミラー対称性では、相関函数(correlation functions)と呼ばれる物理量を計算することに興味がある。[31]相関函数の例のひとつは、弦の相関函数である。A-モデルでは、弦の相関函数を表す、グロモフ・ウィッテン不変量と呼ばれる無限個の数値は計算することが難しい。しかしながら、ミラー対称性は、A-モデルの相関函数をB-モデルの相関函数へ関連付け、カラビ・ヤウ多様体の古典的な複素幾何学へ依存したものとし、より容易に計算を可能とする。この事実がミラー対称性の導入時に数学者たちを興味を惹いた点である。[32]
ミラー対称性の応用
数え上げ幾何学

ミラー対称性の重要な数学への応用の多くは、数え上げ幾何学と呼ばれる数学の分野に属している。数え上げ幾何学では、典型的には代数幾何学を使い、幾何学的な問題の解の数を数え上げることに興味がある。数え上げ幾何学のもっとも早い時期の問題の一つに、ギリシャの数学者アポロニウスによる紀元前200年頃に提案された問題である。彼は、どのようにしたら、与えられた3つの円に接する平面上の円はいくつあるかと問うた。[33] 一般に、アポロニウスの問題の解は、8つの円が存在するである。右の図は黒で示した3つの与えられた円の例を示している。

数学の数え上げ問題はしばしば、多項式の値がゼロとなる点として定義されるいわゆる代数多様体という幾何学的対象のクラスに関係している。例えば、クレブシュ3次曲面は左に図示してある4変数の3次多項式により定義される。19世紀の数学者アーサー・ケイレイ(Arthur Cayley)とジョージ・サルモン(George Salmon)の結果は、この曲面上にはちょうど 27 本の直線があるとことである。[34]
この問題を一般化すると、上に述べたカラビ・ヤウ多様体である5次3次元多様体の上に何本の直線を描くことができるかという問題となる。この問題は19世紀のドイツの数学者ヘルマン・シューベルト(Hermann Schubert)により解かれ、彼はそのような直線はちょうど 2,875 本存在することを発見しました。1986年に幾何学者セルダン・カッツ(Sheldon Katz)が、5次3次元多様体(quintic)に完全に入っている(円のように)次数が 2 の曲線の数は 609,250 個あることを証明しました。[35]
1991年頃には、数え上げ幾何学の古典的な問題の大半が解かれ、数え上げ幾何学への興味は下火になり始めました。数学者マーク・グロス(Mark Gross)によれば、「古い問題が解かれるとともに、人々はシューベルトの数を現代のテクニックを使いチェックするほうへ戻りはしたものの、非常に古めかしいものでした。」[36] この分野は1991年5月にふたたび活発化した。そのとき物理学者であるフィリップ・キャンデラス(Philip Candelas)、ゼニア・デ・ラ・オッサ(Xenia de la Ossa)、ポール・グリーン(Paul Green)とリンダ・パークス(Linda Parks)は、ミラー対称性が5次カラビ・ヤウ多様体に含まれる次数 3 の曲線の数を数えることに使うことができるかもしれないことを示した。大まかにいうと、カラビ・ヤウ多様体の内部に完全に含まれる球として、次数 3 の曲線を考えることができる。[37] キャンデラスと彼の協力者はそのような6次元のカラビ・ヤウ多様体は次数 3 の曲線をちょうど 317,206,375 個含むことができることを発見した。[38]
5次3次元多様体(quintic threefold)上の次数3の曲線を数えることに加えて、キャンデラスと彼の協力者は、数学者たちの得た結果をはるかに超える有理曲線の数え上げに関するより一般的な数多くの結果を得た。[39] この仕事で使われた方法は理論物理学からの数学的には厳密(en:mathematical rigor)ではないアイデアを基礎としていたが、数学者たちはミラー対称性予想のいくつかを数学的厳密(en:mathematical proof)に証明した。特に、ミラー対称性の数え上げ幾何学の予想は、現在では厳密に証明されている。[40]
理論物理学
数え上げ幾何学への応用に加えて、ミラー対称性は弦理論での計算の実行の基本的なツールである。位相的弦理論のA-モデルでは、グロモフ・ウィッテン不変量と呼ばれる無限個の数値により、計算することは極めて難しいが、物理的に興味のある量を表現できる。B-モデルでは、計算が古典的な積分へ還元することができ、非常に容易になる。[41] ミラー対称性を適用し、理論家たちはA-モデルでの難しい計算を、等価であるが技術的にはやさしいB-モデル上の計算へ移し替えができる。従って、これらの計算は、弦理論の様々な物理的過程の確率を決定することに使われる。ミラー対称性は他の双対性と結合されて、一方の理論を別の異なる理論の等価な計算へ移し替える。この方法で別な理論の計算へ外出しすることにより、理論家たちは双対性を使わずには計算が不可能であった多くの量の計算が可能となった。[42]
弦理論以外では、ミラー対称性は、基本粒子を記述するために、物理学者が使う形式である場の量子論の一側面を理解することに使われる。例えば、ミラー対称性は、ゲージ理論の性質を理解することに使われる。ゲージ理論は、基本粒子の標準模型の中に現れ、高度に対称性をもった物理理論である。そのような理論は、近接した背景を伝播する弦から発生し、ミラー対称性はこれらの理論の計算をすることに有用な道具である[43]。実際、このアプローチは、ネーサン・サイバーグ(Nathan Seiberg)やエドワート・ウィッテンにより研究された 4次元の時空の中の重要なゲージ理論の計算の実行に使われ、ドナルトソン不変量(Donaldson invariant)の脈絡での数学に良く似ている。[44] ミラー対称性の一般化として、3次元ミラー対称性(3D mirror symmetry)と呼ばれるミラー対称性もあって、3次元時空の中の場の量子論のペアを関係付ける。[45]
ミラー対称性へのアプローチ
ホモロジカルミラー対称性

弦理論や超重力理論のような関連する理論では、「ブレーン」(brane)が点粒子の考え方の高次元への一般化された物理的対象である。例えば、点粒子はゼロ次元のブレーンと考えることができるのに対し、弦は 1次元のブレーンとして考えることができる。また高次元のブレーンも考えることができる。ブレーンということばは、「メンブレーン」(membrane)ということばから来ていて、2次元のブレーンである。[46]
弦理論では、弦 (物理学)は、(2つの端点を持つ構成となっている)開弦と(閉じたループになっている)閉弦があるようだ。D-ブレーンは、開弦を考えると機に発生する重要なブレーンのクラスである。開弦は時空の中を伝搬し、その端点はD-ブレーンの上にあることを要求される。D-ブレーンの中の文字の "D" は、ディリクレ境界条件(Dirichlet boundary condition)として知られているある数学的条件を導入するという事実から来る。[47]
数学的には、ブレーンは圏(category)の概念を使い記述することができる。[48] これは対象と対象の任意のペアに対して、それらの間の射(morphism)からなる数学的な構造である。大半の例では、対象はある数学的な構造を持っていて(例えば、集合、ベクトル空間や位相空間といった)、射はこれらの構造の間の函数により与えられる。[49] 対象がD-ブレーンで、射が2つのD-ブレーン と の間の射が と の間に伸びた開弦の波動函数であるとも考えられる。[50]
位相的弦理論のB-モデルでは、D-ブレーンのカテゴリは、その上に弦が伝搬するカラビ・ヤウ多様体の複素幾何学から構成される。数学のことばでは、カラビ・ヤウ多様体上の連接層の導来圏として知られている。他方、A-モデルのD-ブレーンのカテゴリは、ミラーであるカラビ・ヤウ多様体のシンプレクティック幾何学から構成される。数学では、これは深谷圏として知られている。[51] マキシム・コンツェビッチのホモロジカルミラー対称性予想は、ある意味でこれらの 2つのブレーンのカテゴリが同値であることを言っている。[52]
SYZ予想
ミラー対称性を理解しようとするもう一つのアプローチは、アンドリュー・ストロミンジャー(Andrew Strominger)、シン=トゥン・ヤウ(en:Shing-Tung Yau)、エリック・ザスロフ(Eric Zaslow)により1996年の論文で示唆された。[53] SYZ予想に従うと、ミラー対称性は複雑なカラビ・ヤウ多様体をより単純なピースへ分解し、これらのピースの上での T-双対を考えることにより理解することができる。[54]
オーバービューのセクションでトーラスを考えたことを思い出すと、このトーラスが2つの円の積とみなすことができた。このことは、(図の中の赤い円として示したように)縦の円(経線)を集めた合併として考えることができることを意味する。これらの円をどのように編成するかという補助的な空間が存在し、この空間自体が円となる(ピンクの円で示した)。この空間はトーラス上で経線の円をパラメトライズすると言われる。上で説明したように、ミラー対称性は経線に作用するT-双対に同値で、半径 から へ変換することとなる。
SYZ予想は、このアイデアをより複雑な6次元カラビ・ヤウ多様体の場合へ一般化した予想である。トーラスの場合のように、6次元カラビ・ヤウ多様体をより単純なピースへ分割することができ、この場合には3-トーラス(トーラスの概念を一般化した3次元の対象)が3-球(球の概念を3次元へ一般化したもの)によりパラメトライズされる。[55] T-双対はこの分解に現れるように、円から3次元トーラスへ拡張が可能で、SYZ予想はミラー対称性がこれらの3次元トーラスのT-双対の同時に適用さることと同値であることを言っている。[56] このようにして、SYZ予想はカラビ・ヤウ多様体の上にミラー対称性がどのように作用するかの幾何学的な素描を与えた。
歴史と発展
ミラー対称性の発見
ミラー対称性のアイデアは、1980年代中期まで遡ることができ、そのときは半径 の円の上の伝搬している弦が物理学的には、適当に計量の単位をとると、半径 の円の上を伝搬している弦と等価であることに気付いたときである。[57] この現象は、現在ではT-双対として知られていて、ミラー対称性に密接に関連していることが理解されている。
1985年からの論文の中で、フィリップ・キャンデラス(Philip Candelas)、ガリー・ホロビッツ(Gary Horowitz)、アンドリュー・ストロミンジャー(Andrew Strominger)とエドワード・ウィッテン(Edward Witten)は弦理論をカラビ・ヤウ多様体上へコンパクト化 (物理学)することで、大まかには理論が素粒子理論の標準モデル(standard model of particle physics)に似たものとなることを示した。[58] この発展につづき、多くの物理学者たちは、弦理論に基礎を持つ素粒子物理の現実に合うモデルを構成できるのではないかと期待し、カラビ・ヤウコンパクト化の研究を始めた。そのような物理的なモデルが与えるには、対応するカラビ・ヤウ多様体が一意に再構成することができないことには注意する必要があった。代わりに、同一の物理から発生する 2つのカラビ・ヤウ多様体が存在することを発見した。[59]
カラビ・ヤウ多様体とある共形場理論(conformal field theory)の間の関係の研究により、ブライアン・グリーン(Brian Greene)とローネン・プレッサー(Ronen Plesser)は、非自明なミラー関係にあることを発見した[60]。さらにこの関係の証拠は、プリップ・キャンデラス(Philip Candelas)とモニカ・リンカー(Monika Lynker)とロルフ・シームリック(Rolf Schimmrigk)の仕事からで結論されていて、彼らは計算機により多くの数のカラビ・ヤウ多様体を研究する中から、それらの中にミラーペアが現れることを発見した[61]。
ミラー対称性の応用が見つかる
数学者たちは1990年頃からミラー対称性に興味を持ち始めた。1990年頃は、物理学者のフィリップ・キャンデラス、ゼニア・デ・ラ・オッサ、パウル・グリーン、リンダ・パークス[62]がミラー対称性を使うと数え上げ幾何学のある問題で、10年以上解けていない問題が解けるであろうことを示した[63]。これらの結果は、1991年のバークレーでの数理科学研究所(Mathematical Sciences Research Institute)(MSRI)での研究集会で提案された。この研究集会の中で、有理曲線の数え上げ問題をキャンデラスの計算した数の一つが、ノルウェーの数学者ゲイル・エリングスラッド(Geir Ellingsrud)とシュタイン・アリルド・シュトローム(Stein Arild Strømme)が見かけ以上に厳密なテクニックを使い得ていた数に不一致であることが認知された。[64] この研究集会で多くの数学者が、キャンデラスの仕事は、厳密な数学的な議論を基礎としていないので、誤っているのではないかとの前提に立っていた。しかしながら、それらの解を試してみると、エリングスラッドとシュトロームは、彼らの行った計算機のコードが誤っていることを発見し、このコードを正しくすると、解がキャンデラスと協力者たちの得ていた解に一致するという答えを得た。[65]
証明されたミラー対称性
1990年、エドワード・ウィッテンは弦理論を簡素化した位相的場の理論を導入し[66]、物理学者たちは位相的場の理論にもミラー対称性のバージョンが存在することを示した。[67] この位相的場の理論についてのステートメントは、普通は数学的な脈絡でのミラー対称性の定義として使われている。[68] 1995年、数学者マキシム・コンツェビッチ(Maxim Kontsevich)は、弦理論の物理的なミラー対称性にアイデアの基礎を置く新しい数学的な予想を提案した[69]。ホモロジカルミラー対称性として知られているこのミラー対称性予想は、ミラー対称性を2つの数学的構造の同値性として定式化した。すなわち、カラビ・ヤウ多様体上の連接層の導来圏とそのミラーの深谷圏の同値性である。[70]
1996年から2000年にかけての、アレクサンダー・ギベンタール(Alexander Givental)、ボング・リアン(Bong Lian)、ケフェング・リウ(Kefeng Liu)、シン=トゥン・ヤウ(Shing-Tung Yau)はコンセビッチのいくつかのアイデアをどのようにして有理曲線の実際の数え上げに精密化して適用することができるかを示した。[71] これらの結果が、現次元での、ミラー対称性の数学的な証明をどのように考えるのかを示している。
関連項目
- 3次元ミラー対称性この日本語化は本記事のノート欄にあります。
- チャーン・サイモンズ理論
- ドナルドソン・トーマス理論
- モンストラス・ムーンシャイン
- サイバーグ・ウィッテン不変量
- 頂点作用素代数
- ウォールクロッシング
脚注
- ^ Yau and Nadis 2010
- ^ Givental 1996, 1998; Lian, Liu, Yau 1997, 1999, 2000
- ^ Hori et al. 2003; Aspinwall et al. 2009
- ^ Zaslow 2008
- ^ Strominger, Yau, and Zaslow 1996
- ^ 入手可能な弦理論の入門書は、Greene 2000 を参照。
- ^ Wald 1984, p. 4
- ^ Zwiebach 2009, p. 8
- ^ a b Yau and Nadis 2010, Ch. 6
- ^ 個の類似は Greene 2000, p. 186 で例として使われている。
- ^ Yau and Nadis 2010, p. ix
- ^ Dixon 1988; Lerche, Vafa, and Warner 1989
- ^ Witten 1990
- ^ Givental 1996, 1998; Lian, Liu, Yau 1997, 1999, 2000
- ^ Zaslow 2008, p.531
- ^ Zaslow 2008, p.523
- ^ Yau and Nadis 2010, p.168
- ^ Hori et al. 2003, p. xix
- ^ Zaslow 2008, p.530
- ^ Zaslow 2008, p.531
- ^ さらに詳しくは、トーラスはモジュラ群に対し基本領域によりパラメトライズされる。
- ^ Zaslow 2008, p.531
- ^ Zaslow 2008, p. 531
- ^ Zaslow 2008, p.531
- ^ Zaslow 2008, p.532
- ^ Zaslow 2008, p.532
- ^ Zaslow 2008, p.533
- ^ Yau and Nadis 2010, p. 160
- ^ Yau and Nadis 2010, p. 161
- ^ Yau and Nadis 2010, p. 163
- ^ Zaslow 2008, p. 529
- ^ Zaslow 2008, p. 534
- ^ Yau and Nadis 2010, p.166
- ^ Yau and Nadis 2010, p.167
- ^ Yau and Nadis 2010, p.166
- ^ Yau and Nadis 2010, p.169
- ^ Yau and Nadis 2010, p.168
- ^ Yau and Nadis 2010, p.169
- ^ Yau and Nadis 2010, p.171
- ^ Yau and Nadis 2010, p.172
- ^ Zaslow 2008, pp. 533–4
- ^ Zaslow 2008, sec. 10
- ^ Hori et al. 2003, p. 677
- ^ Hori et al. 2003, p. 679
- ^ Intriligator and Seiberg 1996
- ^ Moore 2005, p.214
- ^ Moore 2005, p.215
- ^ Aspinwall et al. 2009
- ^ A basic reference on category theory is Mac Lane 1998.
- ^ Zaslow 2008, p.536
- ^ Aspinwall et al. 2009, p.575
- ^ Aspinwall et al. 2009, p.616
- ^ Strominger, Yau, and Zaslow 1996
- ^ Yau and Nadis 2010, p.174
- ^ より詳しくは、3-球の各々の点に付随した3-トーラスが存在する。ただし例外は、特異点を持つトーラスに対応するある悪い性質を持つ点がありうる。Yau and Nadis 2010, pp.176--7を参照のこと。
- ^ Yau and Nadis 2010, p.178
- ^ This was first observed in Kikkawa and Yamasaki 1984 and Sakai and Senda 1986.
- ^ Candelas et al. 1985
- ^ This was observed in Dixon 1988 and Lerche, Vafa, and Warner 1989.
- ^ Green and Plesser 1990; Yau and Nadis 2010, p. 158
- ^ Candelas, Lynker, and Schimmrigk 1990; Yau and Nadis 2010, p. 163
- ^ Candelas et al. 1991
- ^ Yau and Nadis 2010, p.165
- ^ Yau and Nadis 2010, p.169--170
- ^ Yau and Nadis 2010, p.170
- ^ Witten 1990
- ^ Vafa 1992; Witten 1992
- ^ Hori et al. 2003, p. xviii
- ^ Kontsevich 1995
- ^ Kontsevich 1995
- ^ Givental 1996, 1998; Lian, Liu, Yau 1997, 1999, 2000
参考文献
References
- Aspinwall, Paul; Bridgeland, Tom; Craw, Alastair et al., eds (2009). Dirichlet Branes and Mirror Symmetry. American Mathematical Society. ISBN 978-0-8218-3848-8
- Candelas, Philip; de la Ossa, Xenia; Green, Paul; Parks, Linda (1991). “A pair of Calabi–Yau manifolds as an exactly soluble superconformal field theory”. Nuclear Physics B 342 (1): 21–74. Bibcode: 2007CMaPh.276..671W. doi:10.1007/s00220-007-0354-8.[出典無効]
- Candelas, Philip; Horowitz, Gary; Strominger, Andrew; Witten, Edward (1985). “Vacuum configurations for superstrings”. Nuclear Physics B 258: 46–74. Bibcode: 1985NuPhB.258...46C. doi:10.1016/0550-3213(85)90602-9.
- Candelas, Philip; Lynker, Monika; Schimmrigk, Rolf (1990). “Calabi–Yau manifolds in weighted ”. Nuclear Physics B 341 (1): 383–402. Bibcode: 1990NuPhB.341..383C. doi:10.1016/0550-3213(90)90185-G.
- Dixon, Lance (1988). “Some world-sheet properties of superstring compactifications, on orbifolds and otherwise”. ICTP Ser. Theoret. Phys. 4: 67–126. ISBN 978-9971-5-0452-6.
- Givental, Alexander (1996). “Equivariant Gromov-Witten invariants”. International Mathematics Research Notices 1996 (13): 613–663. doi:10.1155/S1073792896000414.
- Givental, Alexander (1998). “A mirror theorem for toric complete intersections”. Topological field theory, primitive forms and related topics: 141–175. doi:10.1007/978-1-4612-0705-4_5. ISBN 978-1-4612-6874-1.
- Greene, Brian (2000). The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory. Random House. ISBN 978-0-9650888-0-0
- Greene, Brian; Plesser, Ronen (1990). “Duality in Calabi–Yau moduli space”. Nuclear Physics B 338 (1): 15–37. Bibcode: 1990NuPhB.338...15G. doi:10.1016/0550-3213(90)90622-K.
- Hori, Kentaro; Katz, Sheldon; Klemm, Albrecht et al., eds (2003). Mirror Symmetry. American Mathematical Society. ISBN 0-8218-2955-6
- Hori, Kentaro; Vafa, Cumrun (2000). "Mirror Symmetry". arXiv:hep-th/0002222。
- Intriligator, Kenneth; Seiberg, Nathan (1996). “Mirror symmetry in three-dimensional gauge theories”. Physics Letters B 387 (3): 513–519. Bibcode: 1996PhLB..387..513I. doi:10.1016/0370-2693(96)01088-X.
- Kikkawa, Keiji; Yamasaki, Masami (1984). “Casimir effects in superstring theories”. Physics Letters B 149 (4): 357–360. Bibcode: 1984PhLB..149..357K. doi:10.1016/0370-2693(84)90423-4.
- Kontsevich, Maxim (1995a). “Enumeration of Rational Curves Via Torus Actions”. The Moduli Space of Curves. Birkhäuser. pp. 335. doi:10.1007/978-1-4612-4264-2_12. ISBN 978-1-4612-8714-8.
- Kontsevich, Maxim (1995b). “Homological algebra of mirror symmetry”. Proceedings of the International Congress of Mathematicians: 120–139. arXiv:alg-geom/9411018. Bibcode: 1994alg.geom.11018K.
- Lerche, Wolfgang; Vafa, Cumrun; Warner, Nicholas (1989). “Chiral rings in superconformal theories”. Nuclear Physics B 324 (2): 427–474. Bibcode: 1989NuPhB.324..427L. doi:10.1016/0550-3213(89)90474-4.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1997). “Mirror principle, I”. Asian Journal of Math 1: 729–763. arXiv:alg-geom/9712011. Bibcode: 1997alg.geom.12011L.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1999a). “Mirror principle, II”. Asian Journal of Math 3: 109–146. arXiv:math/9905006. Bibcode: 1999math......5006L.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (1999b). “Mirror principle, III”. Asian Journal of Math 3: 771–800. arXiv:math/9912038. Bibcode: 1999math.....12038L.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (2000). “Mirror principle, IV”. Surveys in Differential Geometry: 475–496. arXiv:math/0007104. Bibcode: 2000math......7104L.
- Mac Lane, Saunders (1998). Categories for the Working Mathematician. ISBN 978-0-387-98403-2
- Moore, Gregory (2005). “What is ... a Brane?” (PDF). Notices of the AMS 52: 214 2013年6月閲覧。.
- Sakai, Norisuke; Senda, Ikuo (1986). “Vacuum energies of string compactified on torus”. Progress of Theoretical Physics 75 (3): 692–705. Bibcode: 1986PThPh..75..692S. doi:10.1143/PTP.75.692.
- Strominger, Andrew; Yau, Shing-Tung; Zaslow, Eric (1996). “Mirror symmetry is T-duality”. Nuclear Physics B 479 (1): 243–259. arXiv:hep-th/9606040. Bibcode: 1996NuPhB.479..243S. doi:10.1016/0550-3213(96)00434-8.
- Vafa, Cumrun (1992). “Topological mirrors and quantum rings”. Essays on mirror manifolds: 96–119. arXiv:hep-th/9111017. Bibcode: 1991hep.th...11017V. ISBN 978-962-7670-01-8.
- Wald, Robert (1984). General Relativity. University of Chicago Press. ISBN 978-0-226-87033-5
- Witten, Edward (1990). “On the structure of the topological phase of two-dimensional gravity”. Nuclear Physics B 340 (2–3): 281–332. Bibcode: 1990NuPhB.340..281W. doi:10.1016/0550-3213(90)90449-N.
- Witten, Edward (1992). “Mirror manifolds and topological field theory”. Essays on mirror manifolds: 121–160. ISBN 978-962-7670-01-8.
- Yau, Shing-Tung; Nadis, Steve (2010). The Shape of Inner Space: String Theory and the Geometry of the Universe's Hidden Dimensions. Basic Books. ISBN 978-0-465-02023-2
- Zaslow, Eric (2008). “Mirror Symmetry”. In Gowers, Timothy. The Princeton Companion to Mathematics. ISBN 978-0-691-11880-2
- Zwiebach, Barton (2009). A First Course in String Theory. Cambridge University Press. ISBN 978-0-521-88032-9
- Givental, Alexander (1996). “Equivariant Gromov-Witten invariants”. International Mathematics Research Notices 1996 (13): 613–663.
- Givental, Alexander (1998). “A mirror theorem for toric complete intersections”. Topological field theory, primitive forms and related topics: 141–175.
- Kontsevich, Maxim (1995). “Homological algebra of mirror symmetry”. Proceedings of the International Congress of Mathematicians: 120–139.
- Lian, Bong; Liu, Kefeng; Yau, Shing-Tung (2000). “Mirror principle, IV”. Surveys in Differential Geometry: 475–496.
- Mac Lane, Saunders (1998). Categories for the Working Mathematician. ISBN 978-0387984032
- Moore, Gregory (2005). “What is... a Brane?” (PDF). Notices of the AMS 52: 214 2013年6月閲覧。.
- Witten, Edward (1992). “Mirror manifolds and topological field theory”. Essays on mirror manifolds: 121–160.
- Zaslow, Eric (2008), “Mirror Symmetry”, in Gowers, Timothy, The Princeton Companion to Mathematics, ISBN 978-0691118802


































