陰函数定理

数学の特に多変数微分積分学における陰函数定理（いんかんすうていり、英: implicit function theoremは、多項関係を多変数函数（特に実多変数函数）に読み替えることを可能にする基本的な道具である。グラフについて見れば、関係のグラフを函数のグラフとして表すということになる。このとき、関係全域を一つの函数のグラフとして表せるとは限らないが、関係の始域を制限すればそのような函数が取れる。陰函数定理はそのような函数の存在を保証するための十分条件を与えるものである。

定理の主張する所は、方程式 F(x₁, …, x_n; y₁, …, y_m) (= F(x; y)) = 0 が、偏微分に関してある種の緩やかな条件を満足するならば、原理的には（つまり必ずしも既知函数の閉じた形に書くことができるとは限らないが）少なくとも適当な円板上において m-個の各変数 y_i を n-個の変数 x_j に関する式 y_i = f_i (x) として表すことができる。F(x, y) = 0 からこれら陰伏函数 f_i(x)が得られるというのは、幾何学的には y = f(x) の定める超曲面と局所的に一致する F(x, y) = 0 によって定義される軌跡である。

一つの例に基づく導入

単位円は二変数函数 f(x, y) = x² + y² の等位線 f(x, y) = 1 として特定される。点 A の周りで y は函数 y(x) として、具体的には g₁(x) = √1 − x² として表される。他方、点 B の周りではそのような函数をとることはできない。

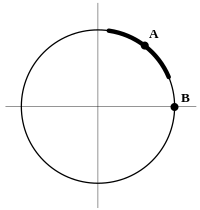
二変数の函数 f を f(x, y) = x² + y² と定めれば、方程式 f(x, y) = 1 はその等位集合 {(x, y)  | f(x, y) = 1} として単位円を描き出す。この単位円を一つの一変数函数 y = g(x) のグラフとして表す方法は存在しない（実際、各 x ∈ (−1, 1) に対して y は二通りの選び方、具体的には ±√1 − x² をとることができる）。

しかしながら、単位円の一部分であれば一変数函数のグラフとして表すことが可能である。いま g₁(x) = √1 − x² (−1 < x < 1) とすれば、y = g₁(x) のグラフは単位円の上半を与える。同様に g₂(x) = −√1 − x² (−1 < x < 1) とすれば、y = g₂(x) のグラフは単位円の下半である。

陰函数定理の目的は、g₁(x) や g₂(x) のような函数の存在を、明示的に式を書き下せないというような状況下でさえ、知ることである。定理が保証することは、g₁(x) や g₂(x) が可微分であること、および可微分であるということが f(x, y) の式が明らかでない場合でも保証されることである。

定理の主張

f: R^n+m → R^m は連続的微分可能とする。始域 R^n+m を直積集合 Rⁿ × R^m と見做して、この直積に属する元を (x, y) = (x₁, …, x_n; y₁, …, y_m) と書く。そのような函数 f が与えられたところから始めて、最終的に函数 g: Rⁿ → R^m でそのグラフ (x, g(x)) がちょうど f(x, y) = 0 を満たす点 (x, y) 全体の成す集合と一致するようなものを見つけることを考える。

既に述べたとおり、そのようなことは常に可能というわけではない。そこで適当な一点 (a, b) = (a₁, …, a_n; b₁, …, b_m) で f(a, b) = 0 を満足するものを固定し、そのような点 (a, b) の近くで目的に合う g を見つけることに視点を移す。すなわち、Rⁿ の開集合 U で点 a を含むものと、R^m の開集合 V で点 b を含むもの、および函数 g: U → V の三つ組 U, V, g で、g のグラフが U × V 上で関係 f = 0 を満足するもの、式で書けば

$\{(\mathbf {x} ,g(\mathbf {x} ))\mid \mathbf {x} \in U\}=\{(\mathbf {x} ,\mathbf {y} )\in U\times V\mid f(\mathbf {x} ,\mathbf {y} )=0\}$

を満足するものを求めたい。

陰函数定理を述べるためには、f のヤコビ行列（函数行列）が必要である。それは f のすべての偏微分によって形作られる行列で、(a, b) における値は

$(Df)(\mathbf {a} ,\mathbf {b} )=\left({\begin{array}{ccc|ccc}{\dfrac {\partial f_{1}}{\partial x_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\dfrac {\partial f_{1}}{\partial x_{n}}}(\mathbf {a} ,\mathbf {b} )&{\dfrac {\partial f_{1}}{\partial y_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\dfrac {\partial f_{1}}{\partial y_{m}}}(\mathbf {a} ,\mathbf {b} )\\\vdots &\ddots &\vdots &\vdots &\ddots &\vdots \\{\dfrac {\partial f_{m}}{\partial x_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\dfrac {\partial f_{m}}{\partial x_{n}}}(\mathbf {a} ,\mathbf {b} )&{\dfrac {\partial f_{m}}{\partial y_{1}}}(\mathbf {a} ,\mathbf {b} )&\cdots &{\dfrac {\partial f_{m}}{\partial y_{m}}}(\mathbf {a} ,\mathbf {b} )\end{array}}\right)=(X\mid Y)$

で与えられる。右辺において、X は変数 x_i たちに関する偏微分からなる行列、Y は変数 y_j に関する偏微分からなる行列である。陰函数定理が述べるのは、このときの行列 Y が正則ならば、所期の通りの U, V, g が存在することである。以上全ての仮定をまとめれば以下の定理を得る。

陰函数定理 ― f: R^n+m → R^m は連続的微分可能で、R^n+m は座標系 (x, y) を持つとする。点 (a, b) = (a₁, …, a_n; b₁, …, b_m) で f(a, b) = c (c ∈ R^m) を満たすものを固定する。このとき、行列 (∂f_i⁄∂y_j(a, b))_i,j が正則ならば、a を含む開集合 U, b を含む開集合 V および一意的な連続的微分可能函数 g: U → V で

$\{(\mathbf {x} ,g(\mathbf {x} ))\mid \mathbf {x} \in U\}=\{(\mathbf {x} ,\mathbf {y} )\in U\times V\mid f(\mathbf {x} ,\mathbf {y} )=\mathbf {c} \}$

を満足するものが存在する。

正則性 (regularity) に関して以下のような一般化が可能である:

1. f に対して U × V の内側で k-階までの導函数が全て連続的微分可能という仮定を追加するならば、定理にいう陽函数 g も同じく U の内側で k-階まで連続的微分可能で、

   ${\frac {\partial g}{\partial x_{j}}}(x)=-\left({\frac {\partial f}{\partial y}}(x,g(x))\right)^{-1}{\frac {\partial f}{\partial x_{j}}}(x,g(x))$

   を満足することが示せる。
2. 解析的陰函数定理: 同様に、f が U × V の内側で解析的ならば、g も U の内側で解析的である

例の再考

ふたたび単位円の例に戻ろう。すなわち、定理において n = m = 1 および f(x, y) = x² + y² − 1 と置いた場合であり、ヤコビ行列は 1×2-行列

$(Df)(a,b)={\begin{pmatrix}{\frac {\partial f}{\partial x}}(a,b)&{\frac {\partial f}{\partial y}}(a,b)\end{pmatrix}}={\begin{pmatrix}2a&2b\end{pmatrix}}$

で与えられる。したがって定理に言う Y はここでは単に数 2b で、それが定める線型写像が正則であるための必要十分条件は b ≠ 0 である。ゆえに陰函数定理によれば、単位円は、y ≠ 0 なる任意の点に対して局所的に y = g(x) の形に書くことができる。しかし、既に上でも述べたが、点 (±1, 0) においては問題が生じる。陰函数定理はこれら二つの点においても適用することは未だ可能であるが、それは x を y の函数 x = h(y) と見てのことである。実際、そのグラフを (h(x), y) とすれば、b = 0 のとき a = 1 と取れるから、局所的にこの形の函数に表されるための条件は満足されている。

y の x に関する陰函数微分、および x の y に関する陰函数微分は、陰函数 x² + y² − 1 の全微分 を 0 に等しいと置いた

$2x\,dx+2y\,dy=0$

から求めることができる。すなわち、dy/dx = −x/y および dx/dy = −y/x が成り立つ。

応用: 座標変換

Suppose we have an m-dimensional space, parametrised by a set of coordinates $(x_{1},\ldots ,x_{m})$. We can introduce a new coordinate system $(x'_{1},\ldots ,x'_{m})$ by supplying m functions $h_{1}\ldots h_{m}$. These functions allow us to calculate the new coordinates $(x'_{1},\ldots ,x'_{m})$ of a point, given the point's old coordinates $(x_{1},\ldots ,x_{m})$ using $x'_{1}=h_{1}(x_{1},\ldots ,x_{m}),\ldots ,x'_{m}=h_{m}(x_{1},\ldots ,x_{m})$. One might want to verify if the opposite is possible: given coordinates $(x'_{1},\ldots ,x'_{m})$, can we 'go back' and calculate the same point's original coordinates $(x_{1},\ldots ,x_{m})$? The implicit function theorem will provide an answer to this question. The (new and old) coordinates $(x'_{1},\ldots ,x'_{m},x_{1},\ldots ,x_{m})$ are related by f = 0, with

$f(x'_{1},\ldots ,x'_{m},x_{1},\ldots x_{m})=(h_{1}(x_{1},\ldots x_{m})-x'_{1},\ldots ,h_{m}(x_{1},\ldots ,x_{m})-x'_{m}).$

Now the Jacobian matrix of f at a certain point (a, b) [ where $a=(x'_{1},\ldots ,x'_{m}),b=(x_{1},\ldots ,x_{m})$ ] is given by

$(Df)(a,b)=\left[{\begin{matrix}-1&\cdots &0\\\vdots &\ddots &\vdots \\0&\cdots &-1\end{matrix}}\left|{\begin{matrix}{\frac {\partial h_{1}}{\partial x_{1}}}(b)&\cdots &{\frac {\partial h_{1}}{\partial x_{m}}}(b)\\\vdots &\ddots &\vdots \\{\frac {\partial h_{m}}{\partial x_{1}}}(b)&\cdots &{\frac {\partial h_{m}}{\partial x_{m}}}(b)\\\end{matrix}}\right.\right]=[-1_{m}|J].$

where 1_m denotes the m × m identity matrix, and J is the m × m matrix of partial derivatives, evaluated at (a, b). (In the above, these blocks were denoted by X and Y. As it happens, in this particular application of the theorem, neither matrix depends on a.) The implicit function theorem now states that we can locally express $(x_{1},\ldots ,x_{m})$ as a function of $(x'_{1},\ldots ,x'_{m})$ if J is invertible. Demanding J is invertible is equivalent to det J ≠ 0, thus we see that we can go back from the primed to the unprimed coordinates if the determinant of the Jacobian J is non-zero. This statement is also known as the inverse function theorem.

例: 極座標系

As a simple application of the above, consider the plane, parametrised by polar coordinates (R, θ). We can go to a new coordinate system (cartesian coordinates) by defining functions x(R, θ) = R cos(θ) and y(R, θ) = R sin(θ). This makes it possible given any point (R, θ) to find corresponding cartesian coordinates (x, y). When can we go back and convert cartesian into polar coordinates? By the previous example, it is sufficient to have det J ≠ 0, with

$J={\begin{bmatrix}{\frac {\partial x(R,\theta )}{\partial R}}&{\frac {\partial x(R,\theta )}{\partial \theta }}\\{\frac {\partial y(R,\theta )}{\partial R}}&{\frac {\partial y(R,\theta )}{\partial \theta }}\\\end{bmatrix}}={\begin{bmatrix}\cos \theta &-R\sin \theta \\\sin \theta &R\cos \theta \end{bmatrix}}.$

Since det J = R, conversion back to polar coordinates is possible if R ≠ 0. So it remains to check the case R = 0. It is easy to see that in case R = 0, our coordinate transformation is not invertible: at the origin, the value of θ is not well-defined.

一般化

バナッハ空間版

Based on the inverse function theorem in Banach spaces, it is possible to extend the implicit function theorem to Banach space valued mappings.

Let X, Y, Z be Banach spaces. Let the mapping f : X × Y → Z be continuously Fréchet differentiable. If $(x_{0},y_{0})\in X\times Y$, $f(x_{0},y_{0})=0$, and $y\mapsto Df(x_{0},y_{0})(0,y)$ is a Banach space isomorphism from Y onto Z, then there exist neighbourhoods U of x₀ and V of y₀ and a Fréchet differentiable function g : U → V such that f(x, g(x)) = 0 and f(x, y) = 0 if and only if y = g(x), for all $(x,y)\in U\times V$.

微分不能函数の定める陰函数

Various forms of the implicit function theorem exist for the case when the function f is not differentiable. It is standard that it holds in one dimension. The following more general form was proven by Kumagai based on an observation by Jittorntrum.

Consider a continuous function $f:R^{n}\times R^{m}\to R^{n}$ such that $f(x_{0},y_{0})=0$. If there exist open neighbourhoods $A\subset R^{n}$ and $B\subset R^{m}$ of x₀ and y₀, respectively, such that, for all y in B, $f(\cdot ,y):A\to R^{n}$ is locally one-to-one then there exist open neighbourhoods $A_{0}\subset R^{n}$ and $B_{0}\subset R^{m}$ of x₀ and y₀, such that, for all $y\in B_{0}$, the equation f(x, y) = 0 has a unique solution

$x=g(y)\in A_{0}$,

where g is a continuous function from B₀ into A₀.

関連項目

• 階数一定定理: 陰函数定理および逆写像定理の一般化

参考文献

• Chiang, Alpha C. (1984). Fundamental Methods of Mathematical Economics (3rd ed.). McGraw-Hill 

• Danilov, V.I. (2001), “Implicit function (in algebraic geometry)”, in Hazewinkel, Michiel, Encyclopaedia of Mathematics, Springer, ISBN 978-1-55608-010-4, http://eom.springer.de/i/i050320.htm .

• Edwards, Charles Henry (1994) [1973]. Advanced Calculus of Several Variables. Mineola, New York: Dover Publications. ISBN 978-0-486-68336-2 

• Fritzsche, K.; Grauert, H. (2002). From Holomorphic Functions to Complex Manifolds. Springer. http://books.google.de/books?id=jSeRz36zXIMC&lpg=PP1&dq=fritzsche%20grauert&hl=de&pg=PA34#v=onepage&q&f=false 

• Jittorntrum, K. (1978). “An Implicit Function Theorem”. Journal of Optimization Theory and Applications 25 (4). doi:10.1007/BF00933522. 

• Kudryavtsev, Lev Dmitrievich (2001), “Implicit function”, in Hazewinkel, Michiel, Encyclopaedia of Mathematics, Springer, ISBN 978-1-55608-010-4, http://eom.springer.de/i/i050310.htm .

• Kumagai, S. (1980). “An implicit function theorem: Comment”. Journal of Optimization Theory and Applications 31 (2). doi:10.1007/BF00934117. 

• Lang, Serge (1999). Fundamentals of Differential Geometry. Graduate Texts in Mathematics. New York: Springer. ISBN 978-0-387-98593-0